Sample of chemistry lab report on activation energy of reactions

      

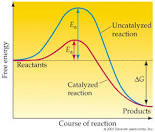
            The activation energy of reaction is the minimum energy needed to initiate a chemical reaction and it is usually designated by Ea. Its SI unit is either in Kilocalories/ mole (Kcal/mole) or KJ/Mole (Kilojoules/mole). A chemical reaction proceeds at an appreciable rate when transitional energy and a good number of molecules present,which are the same or greater than activation energy. In this experiment, the activation of energy for the reaction below was determined:


            The data of rate equation (rate = K [I-][BrO-][H+]2) for this reaction versus temperature (°C) matched Arrhenius equation and gave the approximate activation energy.
In K (T) = -Ea/R + (1/T) + InA (Slope-intercept form)
Or
InK (T2) - InK (T1) = -Ea/R (1/T2 -1/T1), (point-slope form).
         According to Arrhenius Equation, plotting InK versus (1/T) possess a slope (m),which is equivalent to -Ea/R. R= 8.314J/ (Kmole). The activation energy of the reaction was determined by running the reaction at different temperatures (23.7°C, 31.5°C, 43.5°C and 10.2°C). 
Procedure 
         The hot plate was plugged, and water heated to a temperature of 20-25°C on low setting. 250ml of tap water was added into a 400ml beaker and set on the hot plate. Test tubes A and B, which contained appropriate mixtures were prepared and allowed to reach a proper temperature, while swirling a bit for every 3 minutes to ensure consistency of temperature within the test tubes. Data table was prepared for four runs at different temperatures. When temperature stabilized, the stopwatch was prepared and test tubes were removed from the water bath. Solution B was quickly added to solution A while starting the stopwatch immediately as they were mixing, then placing the test tube of the mixture back into the water bath. A Stopwatch was stopped when the familiar color (blue-black) of the reaction was observed and digital thermometer used to determine the temperature of the mixture when the reaction reached completion. The temperature and the time were recorded in the table, and the solutions disposed off in waste bucket. 
         Fresh solutions, A and B, were prepared as earlier and by adjusting the hot plate to temperature of 30-35°C and repeating process, starting from the time the stopwatch was employed in the process until disposal of the solutions. The same was done for temperature 40-45°C. 
     The hot plate was unplugged, followed by pouring out warm water from last trial and replacing it with 250ml cold tap water. Ice was added to the water to reduce its temperature to -10°C, but ice was removed when temperature dropped below -10°C. Two fresh solutions of A and B were prepared and placed in the beaker as the water was cooling down and earlier steps in paragraph one under procedure were repeated when solution cooled to desired temperature (-10°C). 
Results
     The following results were obtained:

Calculation
       The calculations for rate of reactions at different temperature were as indicated below:
Rate = (3.33 X 10-5 M)/dt
For run # 1:
                                     = (3.33 X 10-5M)/ (112 -0) s
                                     = 2.97 X 10-7M/s
For run #2:
                                    = (3.33 X 10-5M)/ (67-0) s
                                    = 4.97 X 10-7M/s
For run 3:
                                    = (3.33 X 10-5M)/ (36-0) s
                                    = 9.25 X 10-7M/s
For run #4:
                                    = (3.33 X 10-5M)/ (283-0) s
                                    = 1.17 X -7M/s
      The calculations for value of K at different temperatures were:
 The K value is given by;
 Rate = K [I-] [BrO-] [H+] 2
 For run #1:
             2.97 X 10-7M/s= K [0.01] [0.04] [0.1]2
K                              = (2.97 X 10-7M/s)/ ([0.01] [0.04] [0.1]2)
                                  = 7.425 X 10-2
For run# 2:
                                  = (4.97 X 10-7M/s)/ ([0.01][0.04][0.1]2)
K                               = 1.2425 X 10-1
For run#3:
               = (9.25 X 10-7M/s)/ ([0.01] [0.04] [0.1]2)
 K                                = 2.3125 X 10-1
For run #4:
                = (1.17 X -7M/s)/ ([0.01][0.04][0.1]2)
 K                                 = 2.925 X 10-2
Table for values of T (K), 1/T (K-1), Rate (M/s), K and InK 

Data Analysis
        Graph of lnk (y-axis) versus 1/T (x-axis, K-1). The plot of InK versus 1/T gave a straight line graph with equation line of y= -5607.4x + 16.28 and with R2 = 0.9992. The slope of the graph represented -Ea/R, which was the activation energy of the reaction.  

Fig1: Graph of ln K versus 1/T (K-1)
        Using the equation of the graph y = y= -5607.4x + 16.28, the activation energy of the reactions was calculated as follows:
        By taking y = mx + C, where m is the slope of the graph and it is equivalent to -Ea/R. Hence m = -5607.4 and m = -Ea/R, R= 8.314J/ (Kmole).
-5607.4            =                      -Ea/8.314J/Kmole;
-Ea                  =                      (-5607.4)/ (8.314J/Kmole);
Ea                    =                      674.4527J/Kmole.  

Amount of time, reaction needed at 60°C.
       Using the equation InK (T2) – InK (T1) = -Ea/R (1/T2 -1/T1), and by taking T1= 43.5°C and T2= 60°C, the value of K would be:
LnK (333) - lnK (316.5)   =      -5607.4(-1.5655X 10-4)
LnK (333/316.5)              =     0.87786                                             
LnK (1.05213)                 =     0.87786
LnK                                  =     [0.87786/1.05213]
K                                     =    e [(0.834364574]
K                                     =     2.30335
The rate of reaction at 60C would be: 
         Rate = K [I-] [BrO-] [H+] 2, but K and concentrations of reaction were known, hence rate was;
                 =      2.30335 [0.01] [0.04] [0.12]
                     =      9.2134 X 10-6M/s
The amount of time needed for the reaction at 60˚C was;
Rate                     =       (3.33 X 10-5 M)/dt
 Dt                       =       (9.2134 X 10-6M/s)/ (3.33 x 10-5M)
                    =       0.276679s 
How increase in temperature affected the reactions.
Chemical reactions increase with increase in temperature and rule of thumb held true for this experiment. As can been seen from the data above, reaction at temperature 31.5°C and 43.5˚C, which were 12°C apart, the time taken for the reaction at 43.5°C(36 seconds) almost doubled the reaction at 31.5°C (67 seconds).
Conclusion
K is constant only for a specific temperature. For various temperatures, K is related to Arrhenius equation as observed in this experiment. It was evidenced that the value of K was directly related to rate of reaction and temperature. For instance, at 43.5°C the K value was 2.3125 X 10-1 while at 10.2°C it was 2.925 X 10-2 .The time taken at 43.5°C was less as compared to time taken at 10.2°C. This indicated that the increase in temperature increased both the K value and the rate of reaction. On the other hand, activation energy was independently related to the rate of reaction and the temperature. Increase in temperature lowered the activation energy while increased the rate of reaction.




 




Comments

Post a Comment

Popular posts from this blog

How to write a Forensic Case Study: Murder of Junko Furuta

Image
Photo of Junko Furuta  For one to score high marks in a Forensic Case Study, one must  adhere to the following: Background of the case: This section contains details about the crime. It includes; the reasons for committing the offense, the conditions which the crime was committed, the circumstances of the crime scene and clear identification of the suspect(s) and the victim(s). One should ensure that the information in this part gives a precise idea of what the case is all about.   Pick and Write an article on Iwriter Website Evidence: This encompasses the piece of evidence found at the crime scene. The evidence should be described in details, that is, ways in which the evidence was collected, processed and preserved.   Forensic Tests: The tests should be two or more that were used to analyze the evidence. The science or theory of instrumentation used must be described fully.   Results: This consists of a detailed explanation of the forensic ...
Read more

Roles of Sodium Carbonate when Extracting Caffeine from Tea Leaves

Image
A student failed to add sodium carbonate (Na 2 CO 3 ) when extracting caffeine from tea leaves with hot water. Though, high yield of caffeine was produced. The crystals melted at temperatures between 202  and 214 degree  Celsius. Explain. This happened because sodium carbonate did not interfere with the caffeine content of the tea leaves. Sodium carbonate (which is a base) deprotonates the phenolic hydroxyl group (-OH) (acidic) of tannins rendering them anionic. Hence, making them highly soluble in water, but parsimoniously soluble in methylene chloride. So, if sodium carbonate is not added when extracting caffeine with hot water, the extracted caffeine would also contain tannins. Additionally, sodium carbonate is added during the extraction to keep caffeine (an alkaloid which is an organic base)  in a free base form. It prevents caffeine from reacting with available acid available, which may lead to the formation cation. It reacts with a phenolic h...
Read more

Arrhenius equation: Increasing temperature increases the rate reaction

Image
The rate of reaction of a particular reaction often increases as one increase the temperature. However, how can this belief be proved using Arrhenius equation? According to the Arrhenius equation, the rate constant (k) is obtained by the ratio of Ea to RT, in which Ea denotes required energy while RT is the available thermal energy. The Arrhenius Equation is given by: k=Ae - (Ea/RT) When Ea is larger, it results in slower reactions. On the other hand, when RT is large, it leads to faster rate of reaction. Larger Ea gives smaller rate constant k, but larger RT produces larger rate constant (k). Larger rate constant increases the rate of reactions.  By using Arrhenius equation, one can easily see that when the temperature is increased, the rate constant will also increase. Let us consider these two scenarios. In the first scenario, Ea=120,000 Joules/mole, R=8.31 mol -1 K -1 , and K=373K.In the second scenario, Ea=120,000Joules/mole, R=8.31 mol -1 K -1 , and K...
Read more